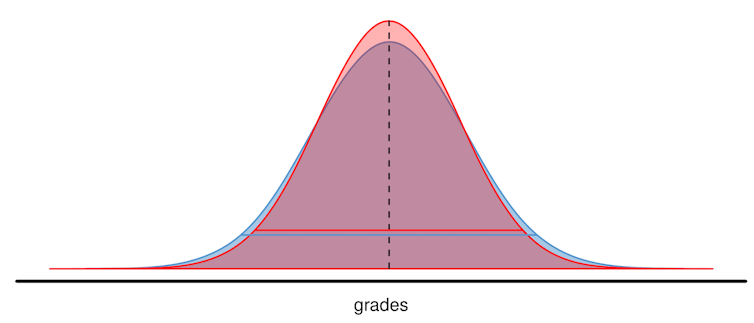
The first was this paper by Rose O'Dea, Malgorzata Lagisz (both UNSW), Michael Jennions (ANU), and Shinichi Nakagawa (UNSW), published in the journal Nature Communications. O'Dea and Nakagawa wrote about the paper in The Conversation late last month. The paper was based on a meta-analysis (an analysis that combined the results from many different studies into a single analysis) of 227 other studies that included over 1.6 million high school students. It tested the 'variability hypothesis' - the idea that male students in STEM (Science, Technology, Engineering, and Maths) subjects show greater variability in performance. That means that there are more male students than female students in each tail of the distribution - more male students than female students do very poorly, and more male students than female students do very well. So, you can think of the distributions of male and female students' grades as something like this:
Overall, girls had significantly higher grades than boys by 6.3%... with 10.8% less variation among girls than among boys...
Girls’ significant advantage of 7.8% in mean grades in non-STEM was more than double their 3.1% advantage in STEM... Variation in grades among girls was significantly lower than that among boys in every subject type, but the sexes were more similar in STEM than non-STEM subjects...In other words, girls had higher grades than boys in both STEM and non-STEM subjects, but the difference in the variability in grades was higher for non-STEM, not STEM, subjects. Here's the resulting distributions:
The ratio of female to male students is equal to one (equal numbers of female and male students) in the top 10% of the distribution of STEM grades, and in the top 2% of non-STEM grades. For the top X% of grades below those thresholds, there are more female than male students (and above those thresholds, there are more male than female students).
Essentially, based on these results, female students outperform male students in both STEM and non-STEM, but they outperform male students by more in non-STEM than in STEM. Which makes these results complementary to those of the second article, by Gijsbert Stoet (Leeds Beckett University) and David C. Geary (University of Missouri), published in the journal Psychological Science (gated, but for the moment at least, this link appears to take you directly to the PDF). Stoet and Geary use PISA (Programme for International Student Assessment) data from over 472,000 students from 67 countries, to look at the intra-individual academic strengths of male and female high school students. Basically, for each student they calculated whether the student performs better (or worse) in reading, maths, or science, compared with the other two subject areas, and by how much better (or worse) they did. They then compare those results between male and female students. They found that:
...there were consistent sex differences in intraindividual academic strengths across reading and science. In all countries except for Lebanon and Romania (97% of countries), boys’ intraindividual strength in science was (significantly) larger than that of girls... Further, in all countries, girls’ intraindividual strength in reading was larger than that of boys, while boys’ intraindividual strength in mathematics was larger than that of girls. In other words, the sex differences in intraindividual academic strengths were near universal...
We found that on average (across nations), 24% of girls had science as their strength, 25% of girls had mathematics as their strength, and 51% had reading. The corresponding values for boys were 38% science, 42% mathematics, and 20% reading.In other words, female students' relative strength was more likely to be in reading, while male students' strength was more likely to be in science or mathematics. They also found that male students were more likely to overestimate their ability in science than female students were.
Alex Tabarrok at Marginal Revolution has the best take on Stoet and Geary's results:
Now consider what happens when students are told. Do what you are good at! Loosely speaking the situation will be something like this: females will say I got As in history and English and B’s in Science and Math, therefore, I should follow my strengthens and specialize in drawing on the same skills as history and English. Boys will say I got B’s in Science and Math and C’s in history and English, therefore, I should follow my strengths and do something involving Science and Math.
Note that this is consistent with the Card and Payne study of Canadian high school students that I disscused [sic] in my post, The Gender Gap in STEM is NOT What You Think. Quoting Card and Payne:
"On average, females have about the same average grades in UP (“University Preparation”, AT) math and sciences courses as males, but higher grades in English/French and other qualifying courses that count toward the top 6 scores that determine their university rankings. This comparative advantage explains a substantial share of the gender difference in the probability of pursing a STEM major, conditional on being STEM ready at the end of high school."
and myself:
"Put (too) simply the only men who are good enough to get into university are men who are good at STEM. Women are good enough to get into non-STEM and STEM fields. Thus, among university students, women dominate in the non-STEM fields and men survive in the STEM fields."So, even though female students may be better than male students in STEM subjects at school, we may see fewer of them studying those subjects at university (let alone taking them as a career), because female students are also better in non-STEM subjects at school, and they are better by more in non-STEM than in STEM, compared with male students. Economists refer to this as the students following their comparative advantage. Female students have a comparative advantage in non-STEM, and male students have a comparative advantage in STEM subjects. That is different from absolute advantage (which female students appear to have in both subject areas, at least according to O'Dea et al. - the gender differences in average results are not consistently significant in Stoet and Geary). The O'Dea et al. results and the Stoet and Geary results both support this comparative advantage interpretation.
One final result from Stoet and Geary still needs further exploration. They report that:
...the relation between the sex differences in academic strengths and college graduation rates in STEM fields is larger in more gender-equal countries.That is a surprising result. If we thought that making genders more equal would reduce the gender gap in STEM, we would expect the exact opposite result! Stoet and Geary's proffered explanation for this seems particularly weak:
Countries with the highest gender equality tend to be welfare states (to varying degrees) with a high level of social security for all its citizens; in contrast, the less gender-equal countries have less secure and more difficult living conditions, likely leading to lower levels of life satisfaction...So, because people feel more secure in countries with better welfare states, which are also the countries with higher gender equality, they are less likely to pursue the high-risk, high-reward jobs in STEM fields, than safer jobs in non-STEM fields. That definitely needs more follow-up research.
Read more:
No comments:
Post a Comment